Magnetic Field Due to a Current Carrying Conductor

Biot-savarts law does a common alteration of-

Current I is following along the path ABCD, along the four edges of the cube (figure-a) creates a magnetic field in the centre of the cube of ${\text{B}}_0$. Find the magnetic field B created at the centre of the cube current I following along the path of the six edges ABCDHEA figure - b

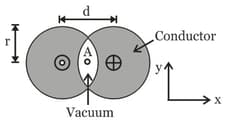
Two long conductors are arranged as shown above to form overlapping cylinders, each of radius r, whose centres are separated by a distance d. Current of density J flows into the plane of the page along the shaded part of one conductor and an equal current flows out of the plane of the page along the shaded portion of the other, as shown. What are the magnitude and direction of the magnetic field at point A ?

Two very long straight parallel wires, parallel to $y$-axis, carry currents $4I$ and $I$, along $+y$-direction and $-y$-direction, respectively. The wires pass through the $x$-axis at the points $(d, 0, 0)$ and $(-d, 0, 0)$ respectively. The graph of magnetic field $z$-component as one moves along the $x$-axis from $x=-d$ to $x=+d$, is best given by

Equal current i is flowing in three infinitely long wires along positive x, y and z directions. The magnitude field at a point (0, 0, -a) would be:

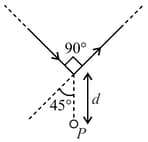
Find the magnetic field at $P$ due to the arrangement shown.

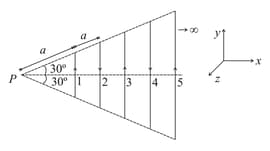
Infinite number of straight wires each carrying current I are equally placed as shown in the figure. Adjacent wires have current in opposite direction. Net magnetic field at point P is.

Two mutually perpendicular conductors carrying currents $I_1 \text{and} I_2$ respectively, lie in one plane. Locus of the point at which the magnetic induction is zero, is a:

Two concentric coils $X$ and $Y$ of radii $16 \mathrm{cm}$ and $10 \mathrm{cm}$ lie in the same vertical plane containing $N-S$ direction. $X$ has $20$ turns and carries $16 \mathrm{A}$. $Y$ has $25$ turns & carries $18 \mathrm{A}$. $X$ has current in anticlockwise direction. The magnitude of net magnetic field at their common centre is-

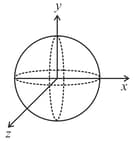
Three rings, each having equal radius R, are placed mutually perpendicular to each other and each having its centre at the origin of co-ordinate system. If current I is flowing through each ring then the magnitude of the magnetic field at the common centre is

$3$ infinitely long thin wires each carrying current $i$ in the same direction, are in the $x-y$ plane in a gravity-free space. The central wire is along the $y$-axis while the other two are along with $x=\mp \mathrm{d. }$If the central wire is displaced along the $z$-direction by a small amount & released, the wire executes the simple harmonic motion. If the linear density of the wire is $\lambda$, find the frequency of oscillation.

Three infinitely long thin wires each carrying current $i$ in the same direction, are in the x-y plane a gravity-free space. The central wire is along the y-axis while the other two are along $x=\mp d$ Find the locus of the points for which the magnetic field B is zero.

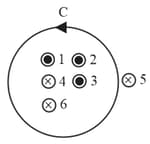
Six wires of current ${\mathrm{I}}_1=\mathrm{1 }\mathrm{A},{\mathrm{I}}_2=\mathrm{2 }\mathrm{A},{\mathrm{I}}_3=\mathrm{3 }\mathrm{A},{\mathrm{I}}_4=\mathrm{1 }\mathrm{A},{\mathrm{I}}_5=\mathrm{5 }\mathrm{A}$ and ${\mathrm{I}}_6=\mathrm{4 }\mathrm{A}$ cut the page perpendicularly at the points $\mathrm{1,\; 2,\; 3,\; 4,\; 5}$ and $6$ respectively as shown in the figure. Find the value of integral $\oint \overrightarrow{B}.d\overrightarrow{l}$ around the circular path $\mathrm{C.}$

A ring of the radius $R$ is placed in the $y-z$ plane and it carries a current $i$. The conducting wires which are used to supply the current to the ring can be assumed to be very long and are placed as shown in the figure. The magnitude of the magnetic field at the centre of the ring $\mathrm{A}$ is

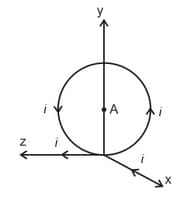
Find the magnetic induction at point $O$, if the current carrying wire is in the shape shown in the figure.

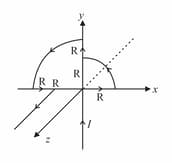
Find the magnetic induction at the origin in the figure shown.

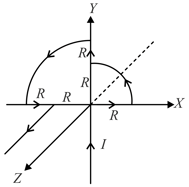
Find the magnetic induction at the origin in the figure shown.

Two circular coils A and B of radius $\frac{5}{\sqrt{2}}$ cm and 5 cm respectively current 5 Amp. and $\frac{5}{\sqrt{2}}$ Amp. respectively. The plane of B is perpendicular to plane of A their centres coincide. Find the magnetic field at the centre.

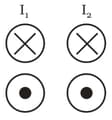
A system of long four parallel conductors whose sections with the plane of the drawing lie at the vertices of a square there flow four equal currents. The directions of these currents are as follows : those marked $\otimes$ point away from the reader, while those marked with a dot point towards the reader. How is the vector of magnetic induction directed at the centre of the square ?

A long straight wire carries a current of $10 \mathrm{A}$ directed along the negative $y-$axis as shown in the figure. A uniform magnetic field $B_0$ of magnitude ${10}^{-6} \mathrm{T}$ is directed parallel to the $x-$axis. What is the resultant magnetic field at the following point $\left(\mathrm{x}=2 \mathrm{m}, \mathrm{z}=0\right)$?